Golden angle
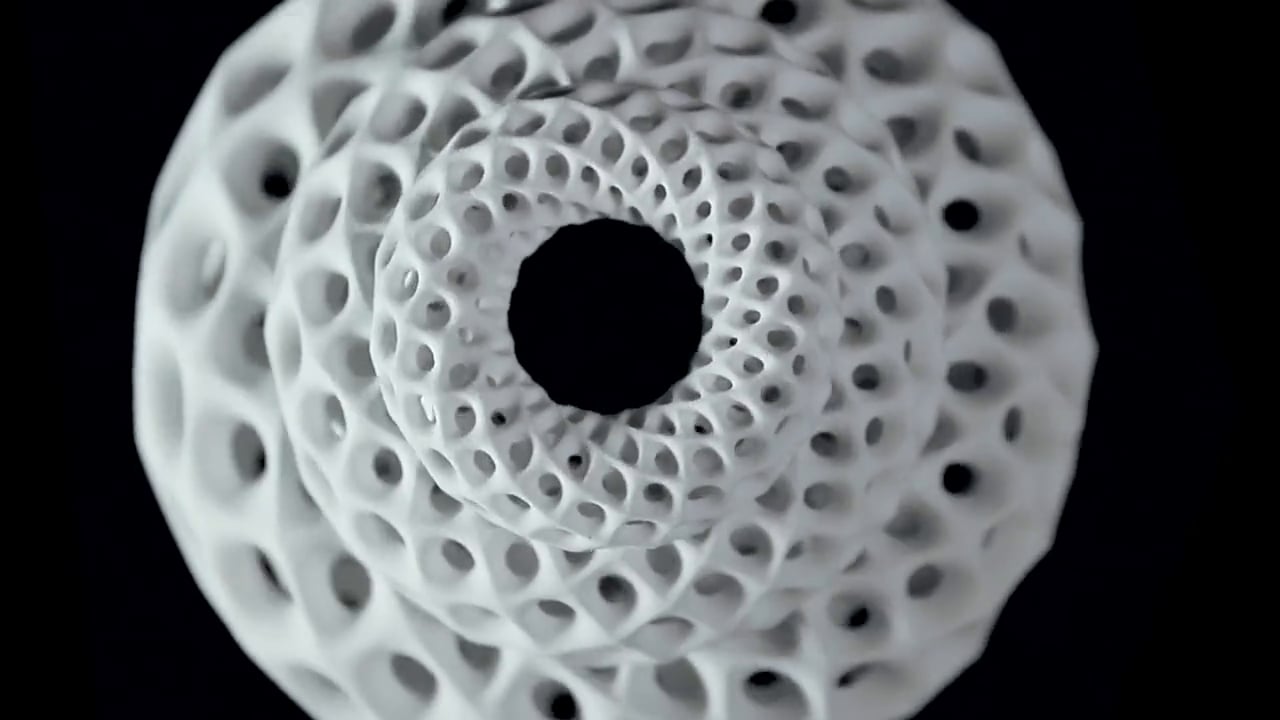
Blooms are 3D printed sculptures designed to animate when spun under a strobe light. Unlike a 3D zoetrope, which animates a sequence of small changes to objects, a bloom animates as a single self-contained sculpture. The bloom’s animation effect is achieved by progressive rotations of the golden ratio, phi (ϕ), the same ratio that nature employs to generate the spiral patterns we see in pinecones and sunflowers. The rotational speed and strobe rate of the bloom are synchronized so that one flash occurs every time the bloom turns 137.5º (the angular version of phi). Each bloom’s particular form and behavior is determined by a unique parametric seed I call a phi-nome (/fī nōm/).
For this video, rather than using a strobe, the camera was set to a very short shutter speed in order to freeze individual frames of the spinning sculpture.


Contains casual footage of a variety of blooms, including some new designs; also demonstrates the use of internal lighting (sometimes in conjunction with external lighting).

Through the use of internal stops, each arm is constrained to rotate a maximum of 68.75° (1/2 the Golden Angle) relative to its neighboring layer.
The Helicone is available at a number of retail venues including the MoMA Design store and Guggenheim Museum store in New York City. It may be found Online at momastore.org, and Amazon.com.
The Helicone is featured in an article in the New York Times.
Materials: laser-cut plywood, brass, beechwood

The Lollipopter is a multicolored, plastic version of the Helicone.
The Lollipopter is available at a number of retail venues. It may also be found Online at Amazon.com.
Materials: polypropylene, stainless steel, ABS
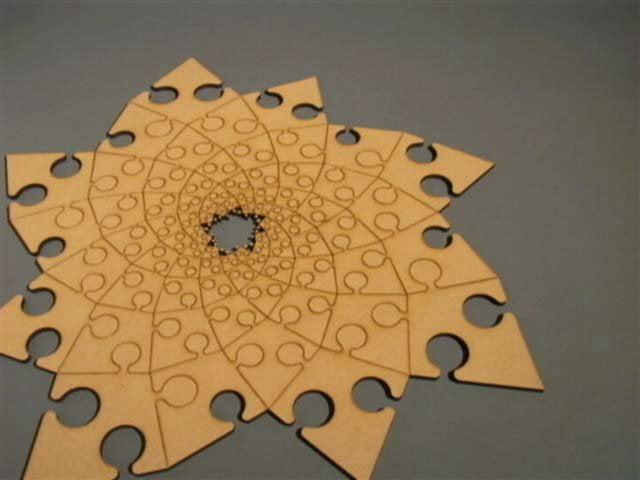
A self-similar tiling, in which every piece is a unique size, but all pieces are the same shape. Each piece is added/removed at an angle of ~137.5 degrees from the previous. This angle—known as the Golden Angle—is manifest in natural objects such as pine cones and sunflowers.
Material: laser-cut MDF
Templates for cutting this tiling may be found at Instructables.com

The transformations in this tower result entirely from rotating the individual layers by the golden angle with respect to their neighboring layers. (Note: this is not CGI; it is a stop-motion animation of laser-cut MDF)
Material: laser-cut MDF

Through the use of internal stops, each layer in this tower is constrained to rotate a maximum of 137.5° (the Golden Angle) relative to its neighboring layer.
Material: laser-cut plywood

The transformations in this tower result entirely from rotating the individual layers by the golden angle with respect to their neighboring layers.
Material: laser-cut plywood

The transformations in this tower result from Incremental Drift, wherein each layer rotates slightly faster than the layer beneath it. There are 32 layers in this tower, so the top layer must make 32 complete revolutions before the tower returns to the same state.
Internal mechanism designed and fabricated by Paul Stepahin.
Material: laser-cut plywood

Through the use of internal stops, each layer in this tower is constrained to rotate a maximum of 137.5° (the Golden Angle) relative to its neighboring layer.
Material: laser-cut plywood


Naturally Occurring Blooms
The plant forms below share the same essential growth process wherein each new leaf or areole is placed 137.5º - the golden angle - around the center from the previous. Any plant that uses this growth process is a potential candidate for being animated as a bloom. Nature uses this same growth process in a wide variety of plant forms, including pine cones, and sunflowers. If you count the number of spirals on any of these plants you will find that they are always Fibonacci numbers.

Here we see an artichoke spinning while being videotaped at 24 frames-per-second with a very fast shutter speed (1/4000 sec). The rotation speed is chosen to cause the artichoke to rotate 137.5º—the golden angle—each time a frame is captured, thus creating the illusion that the leaves are moving up or down the surface of the artichoke. (The movement is wobbly because the artichoke is not completely symmetrical.)
Camera & editing: Charlie Nordstrom

For each frame of the animation the cactus is rotated 137.5º—the golden angle—which creates the illusion that the areoles are emerging from the center of the cactus. (The irregularity of the movement is due to the areoles not being perfectly consistent in their placement.)

For each frame of the animation the cactus is rotated 137.5º—the golden angle—which creates the illusion that the areoles are emerging from the center of the cactus. (The irregularity of the movement is due to the areoles not being perfectly consistent in their placement.)

For each frame of the animation the cactus is rotated 137.5º—the golden angle—which creates the illusion that the areoles are emerging from the center of the cactus. (The irregularity of the movement is due to the areoles not being perfectly consistent in their placement.)
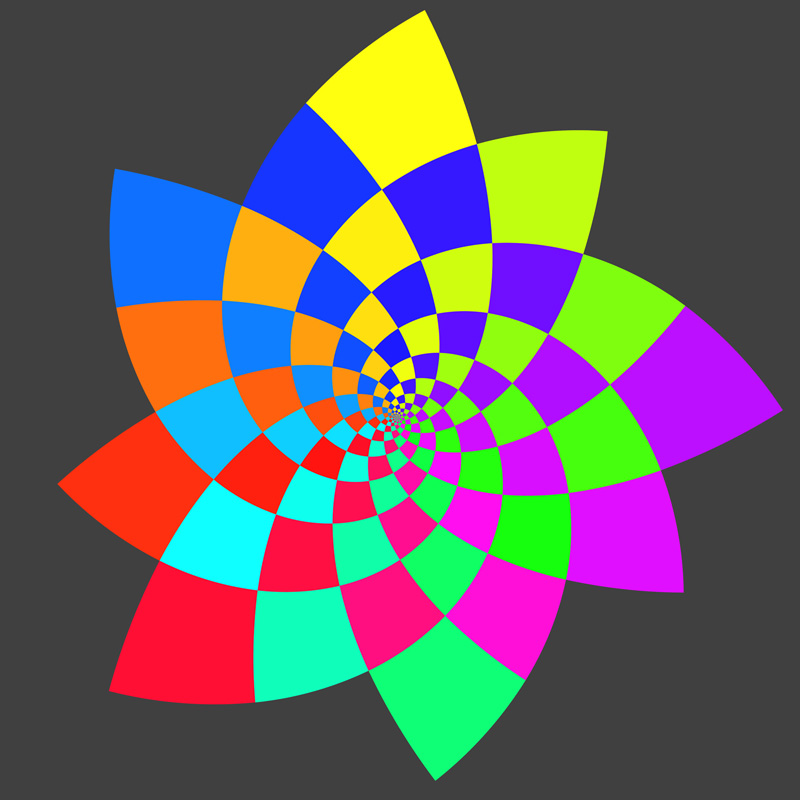
Chromataxis 8x13 H89
The spiral mesh consists of 8 spirals in one direction and 13 spirals in the other. Such a configuration cannot be colored like a checkerboard; a conflict would arise that forces some identically colored cells to abut. To get around this problem, the cells have been colored so that that their hues shift according to their angle around the center. The result is a checkerboard pattern that uses complementary hues to create contrast between cells.
